Photographs: Uttam Ghosh/Rediff.com IMS Learning Resources
The Quantitative Ability and Data Interpretation and Logic sections of the CAT have always been worrisome -- non-engineers or test-takers without math in 10+2/ graduation dread these for two reasons:
- A virtual alienation from math post matriculation and therefore the subject being a once-upon-a-time pursuit
- A fear of comparative disadvantage precipitating from the perception that their engineer counterparts are more equipped to score in these sections.
Engineers on the other hand, have had different reasons for not being treated well by these sections:
- Overconfidence, stemming from the fact that their quantitative and data analysis skills have been well validated by the engineering curriculum and that this is merely an extension of the same
- Emotional anchoring to questions which are prima facie simple, but end up consuming proportionately more time, and therefore ruffle the numeric equilibrium of the engineers.
The above is only an attempt to rebuff a correlation between the test takers' performance in this section and their academic background. This has been further validated by the fact that the IIMs have opened this test to students of all graduation streams.
The CAT is not a test of one's ability to apply mathematical formulae or to mug up the theorems therein, but a test of management skills.
...
Have you aced the CAT? Do you have tips that could help students improve their scores or stress-busting strategies to beat pre-CAT nerves? Send in your advice and experiences to getahead@rediff.co.in with the subject 'My CAT tips' and we'll publish your strategies right here on rediff.com.
Quant and DI modules decoded
Photographs: Dominic Xavier/Rediff.com
Let us try to understand this contextually, starting with the Quant section, with the module which is apparently a test-taker's delight -- Arithmetic. This module is replete with questions on numbers and most of them are based on the principle of symmetry, typically questions on divisibility (eg what is the remainder when 784 is divided by 342? CAT '99) and series (eg what is the 288th term of the series a,b,b,c,c,c,d,d,d,d,e,e,e,e,e? CAT 2003, cancelled version).
The analogy is that symmetry is a widely applicable management concept and facilitates a better understanding of things, processes and even human behaviour.
The next module in the section is Algebra, where the age-old topic, "Time, Speed & Distance", decides the flavour. Questions in this topic are based on the correlation between three variables -- time, speed and distance -- mathematically configured as Distance = SpeedxTime.
The analogy to management is that managers are multitaskers and they have to correlate multiple tasks efficiently and effectively. The students' dexterity in solving questions on this topic is a basic indicator of their ability to multitask.
Geometry, the next module, mirrors your ability to understand space. In fact, managers operate in a very dynamic environment and battles are fought in "markets". Students' ability to understand geometry enhances their connect with spatial configuration, and different points in the XY plane merely replicate the diverse players in the "market".
Most interestingly, is the last module -- Modern Math -- where the popularly voted "grey topic" -- Permutations & Combinations -- is a significant contributor. This topic has the capacity to unleash questions requiring an ability to explore a wide spectrum of possibilities.
The analogy to management is that this ability measures one's capacity for lateral and 'out of the box' thinking, which is a huge upside for success in today's world.
Next is the "Data Interpretation & Logic" section, with three identified test areas over the years -- data interpretation (DI), data sufficiency (DS) and logical reasoning (LR). Questions in DI span a range of statistical tools like line charts, bar graphs, data tables or a combination thereof.
These questions do not just mirror one's numerical aptitude but are designed to test one's data comprehension and data processing ability, which in turn authenticates a range of managerial skills like speculation, extrapolation, strategy formulation, positioning in a cluttered space etc.
DS is an area which validates ones's ability to take decisions on the basis of least available data, a trait which measures a managers's efficiency in a "pace dominated" business world. LR questions test one's ability to grasp complex situations and solve problems in a strategic and effective manner, a skill which empowers an individual to tactfully handle real life business situations.
In a nutshell, these two sections are overtly a test of one's quantitative and data analysis skills, but covertly and more importantly, an indicator of one's "managerial quotient". One needs to develop a manager's approach to connect with the questions in these sections, and not merely look at them from a "math perspective".
The following questions exemplify the approach suggested above...
Question 1
Test takers tend to have a favourable disposition towards this question due to its short length and apparently easy constitution, which invariably puts it in the category of "attempted questions".
However, as one starts putting things in a perspective, the ambiguity quotient creeps in and gradually builds up due to the mismatch between the number of variables and the number of equations, which further intensifies as one is forced to drift towards the hit and trial approach required to solve the question.
However, smart test takers will be able to establish a more efficient connect with the question, as explained below:
The first step is to translate the data into its corresponding equation:
A! + B! + C! = 100A + 10B + C
Having done this, a careful scrutiny reveals that none of A, B and C can take a value of 7 or more as any such value will result in a four-digit number, whereas ABC is given to be a three-digit number. For example, factorials of 7, 8 and 9 are outrightly four-digit numbers; factorial of 6, despite being a three-digit number (720), introduces a "7" in the equation, which is overruled, as the equation can not have a 7, 8 or 9. Thus, even 6 is not a permissible value of these variables.
At this juncture, one can comfortably strike out any option which projects 6, 7, 8, 9 as a value of any of these variables. Thus options a) and b) are eliminated, thereby narrowing down the options to two. Please appreciate that the efforts need to be contextual; had there been three options showcasing values equal to/more than 6, the fourth option would have qualified as the answer. In this case, there are two options with values less than 6, hence the need for the next level of analysis.
Here, one needs to appreciate that the maximum value of ABC can be 3! + 4! + 5!, which is 150 (150 itself is ruled out as 1! + 5! + 0! is not equal to 150). As the number ABC is a three-digit number less than 150, the digit at the hundred's place (ie A) has to be 1.
Further, the inclusion of 5 in the system is mandatory, since it is not possible to get a three-digit number with any permutation of 0, 1, 2, 3, 4 in the given equation. Moreover, 5 can not take the ten's place as that will result in the number being greater than 150.
Factoring in these observations, the above equation changes to a one variable-one equation relationship, which is definitely more manageable:
1! + B! + 5! = 100*1 + 10B + 5 = 105 + 10B
Having decided on the values of A and C as 1 and 5 respectively, the consideration set for B condenses to (0, 2, 3, 4), which after a preliminary "hit and trial" yields 4 as the final value, and the equation eventually configures to:
1! + 4! + 5! = 145. Hence the answer is option c).
Question 2
The first reaction to this question is that of aversion and immediate abdication, for the following obvious reasons:
- The question is apparently from the topic Time, Speed & Distance; a topic that has the capacity to unleash a vast diversity of questions, most of them based on elaborate permutations of meeting possibilities of trains moving on parallel/ same tracks.
- The length of the question is a significant repellant itself. The sheer bulk of the question is a deterrent and keeps the test-taker at bay as the latter is aware of the clock ticking away relentlessly and is responding to the questions under a multitude of mundane pressures!
- The question has a unique pictorial imagery around it and requires the student to flowchart and visualise, which is an added burden and can be avoided as there is a lot of "implied" choice in the selection of questions.
- An inclusion of "none of these" as an option limits the scope of the smart test-taker to arrive at the answer via the "options' route".
Given this background, the evasive test-taker seeks respite in shorter questions or questions from conceptually simpler topics. We take a look at some of the ways this could be solved:
1st method (conventional)
Let the cat be located initially at point C, let the length of the tunnel be "x" kms and let the train be at a distance of "y" km from point A.
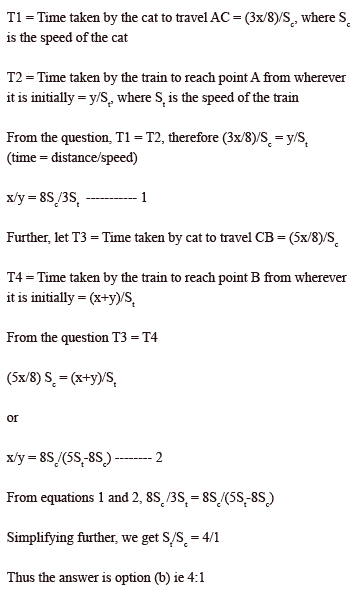
The above method definitely measures up well on the effectiveness scale, but consumes proportionately more time as compared to the following two methods.
2nd method
This is the approach taken by the test-taker who explores various options and zeroes in on the one which satisfies the dynamics of the basic "time-speed-distance" equation.
For example, let us try to validate the second option (which is the answer), which if satisfied, implies that the ratio of the speed of the train to that of the cat is 4:1. Thus, if the speed of the train is 4km/hr, then that of the cat is 1km/hr and if the length of the tunnel is 8 km (recommended as the cat is at a distance which is 3/8 of the length of the tunnel from point A), then the cat is at a distance of 3 km from point A.
Thus, the cat covers the distance AC (C is the point where the cat is initially located) in 3 hours and in this time the train will cover 12 km (which also gives us the initial distance between the train and point A). Now if the cat runs towards point B, then the distance covered by the train will be 20 km (12+8) at a speed of 4 km/hr and that covered by the cat will be 5 km (CB) at a speed of 1 km/hr, both resulting in 5 hr as the time duration.
Hence this option is the answer (other options will yield different time spans for the cat and the train to cover the above mentioned distance segments).
3rd method
This is the manager's approach and definitely yields higher efficiency as compared to other approaches. As in the previous approach, let the length of the tunnel be 8 km, then the cat is at a distance (AC) of 3 km from point A. If the cat runs towards point A, it implies that during the time the cat covers 3 km, the train reaches point A from wherever it is at the onset.
Now if the cat runs towards point B, when it covers 3 km towards point B, the train enters the tunnel (it reaches point A), which means that in order to catch the cat at point B, the train has to traverse the entire length of the tunnel AB (ie 8 km), while the cat has to cover 2 km. This further implies that during the time the train covers 8 km, the cat covers 2 km.
Since time is constant, distance and speed will be in direct proportion. Thus the ratio of the distance covered will be the same as that of the respective speeds. Hence the answer is option (b) ie 4:1.
Question 3
This question has overtly been sourced from the topic Time, Speed & Distance, and evaluates students on intricate concepts of this three-pronged concept area. Covertly, it poses challenges of understanding the correlation between the three variables and configuring them into a relationship in the context of the given question, which is also indicative of an individual's ability to multitask, a potent management trait.
Further, the presence of data pertaining to all three variables (5 and 10 km/hr, 20 km and 1minute) gives the students false leads to work on and tests them on data sufficiency skills, which discriminates the efficient and speedy managers from their inefficacious counterparts.
For example, a dominant proportion of CAT 2004 test-takers focused its efforts on the initial distance of separation between the boats (20 km) and started working on this snippet of data, as shown below:

The above method is inappropriate in the given situation. The smart test-taker will be able to look beyond the redundancy of "20 km" and connect with the fact that the solution manifests in a simple arithmetic calculation, as shown below:
Required distance (ie one minute before they collide) = Relative Speed x Time = 15 km/hr x 1 minute = 15 x(1/60) = 1/4 km
Students need to appreciate the fact that the question merely requires the distance equivalent of 1 minute (given that the relative speed of the objects is 15 km/hr), and a basic caution of upholding the compatibility of the units of the three variables. Getting entrapped in the redundancy of data is also to be watched out for. Given this background, this question surely qualifies as a potential pick!
Question 4
This question is a delight for the test-taker for the following reasons:
- It is apparently based on the concept of "Ratios and Proportions" (module algebra), where the comfort levels are proportionately more.
- It is a short question and in a situation where the clock is ticking away relentlessly, length assumes the proportions of a significant parameter for selecting questions.
- All variables are configured in single degree and the expression is devoid of surds/irrational numbers. Test-takers are normally averse to such inclusions in the question!
Given the above factors, this question extends an invite to the test-taker. This is exactly what happened to a large populace of CAT 2004 takers.
The first milestone can be reached at by an instant application of the law of equality of ratios (if a/b= c/d= e/f, then each ratio is equal to the ratio of the sum of numerators to the sum of denominators) and hence in the given question, "r" will take the value of (a+b+c) /2 (a+b+c), which is equal to 1/2. Hence 1/2 will definitely be a part of the answer. Another way of doing so is a careful scrutiny of the given expression to infer that in an eventuality of a, b and c being equal, each ratio takes the value of 1/2; this approach being a more efficient one as compared to the former.
Having decided that 1/2 qualifies as an answer, the time-pressed test-taker tends to impulsively latch on to this option and chooes option 1. Furthermore, the options are arranged in a sequence, starting with as the first option, thereby propelling the test-taker to anchor on to this obvious option (the human mind is more conditioned to explore options in a symmetrical and ascending order).
However, the seasoned test-taker will look beyond the temptation of marking the obvious and vigilantly truncate options 2 and 4 (which don't reflect as a value), narrowing down the choice of the right option to either option 1 or option 3; if -1 gets validated, the answer is option 3, otherwise it is option 1.
The task, thus, boils down to checking the feasibility of -1, where in there are again two ways. The first one is the algebraic route, where one can take the first two ratios, cross multiply and get the value of (a + b), which can be then plugged in the third ratio to get -1, as shown below:
a / (b + c) = b / (c + a)
ac + a2 = bc + b2; a2 - b2 = -c (a - b); (a - b) (a + b) = -c (a - b); a + b = -c
The other way is the top-down approach, where you start with the assumption that -1 is a possible value and therefore each ration is equal to -1; one of the ways in which this is possible is when each denominator is a conjugate of the numerator i.e in the first ratio, (b + c) = -a; in the second ratio, (c + a) = -b; and in the third ration, (a + b) = -c. In each of these, however, the commonality is that a + b + c = 0. Hence, when the sum of the three variables is equal to zero, "r" will be equal to -1.
Thus, while overtly the question is a test of mathematical skills, covertly and more importantly, it is test of managerial acumen the ability to manage impulse and not seek instant gratification while taking decisions!
Conclusion
Photographs: Uttam Ghosh/Rediff.com
This is an attempt to reiterate the objective of the CAT -- to find candidates with an aptitude for management and an ability to solve problems effectively and efficiently. However, this does not take the focus away from concepts. Not knowing a concept can be a huge deterrent in effectively tackling a question; for example, it is virtually impossible to solve a question on "cyclic quadrilateral" without knowing what is one.
Hence it is suggested to brush up the fundamentals of various modules Arithmetic, Algebra, Geometry and Modern Math. It has been observed that a thorough awareness wrt major concepts can take a test-taker to a performance level of 75 percentile in the CAT. Thereafter the focus needs to shift towards developing an ability to apply concepts and ingrain a problem-solving approach, with a skill to identify the sequential steps for effectively handling the questions.
Students also need to stress on an integrated approach to solving questions; for example, a question might require an application of multiple concepts and therefore demand the proficiency to apply concepts in an integrated manner previous CAT questions reveal that certain questions on time, speed and distance also entailed an application of concepts from geometry, while questions on permutations and combinations required concepts from number theory as well.
An optimum number of section wise tests followed by in-depth analysis is the way forward in this level of preparation. It has been observed that a strong application orientation can take a test-taker to a performance level of 95 percentile in the CAT.
Lastly, it is advisable to take an adequate mix of paper-based and computer-based tests; while paper-based tests gauge your ability to respond to test questions in a conventional way, computer-based tests mirror your adaptation to the new mode of test taking and ensure desired comfort levels therein.
Further, each test needs to be analysed well the attempted questions need to be revisited from a perspective of bettering the approach and reducing the time allocated for solving them; while the unattempted questions need to be explored from a feasibility perspective, which when attempted could have reduced the opportunity cost and apportioned out more time for other questions (the flowchart below details the action plan for an in-depth test analysis).
Optimal test-taking can ensure competence in significant test-taking skills like time management, strategy formulation, prioritization, judicious management of diverse sections, and can help the test-taker reach the 100 percentile!








Comment
article